Help to Conduct Power Analysis in Research
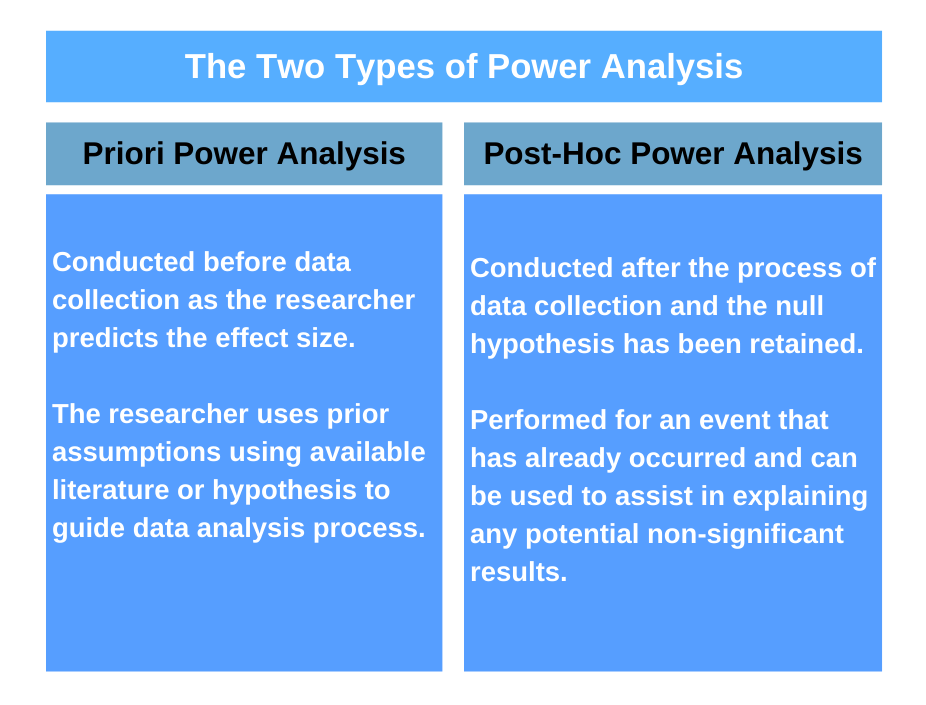
What is Power Analysis?
Power analysis is a statistical tool used in designing and planning studies and research. It is mostly used in biomedical, psychosocial, and business research. Power analysis shows the probability of an effect of interest, type 1 and II errors, significance level when comparing population mean, and hypothesized difference in a t-test.
Power is the probability of suitably discovering a given present difference or effect as significant. Statistical power is equal to (1-ᵦ), which represents the sensitivity of a test, and the power of a test is set at 80%. The power of a test (ᵦ) depends on sample size (N), population effect size (ES), and decision criteria (α).
Power analysis is important in planning future studies, interpreting a negative trial without a significant effect, and reducing the rate of data interpretation errors. Power analysis can be performed using statistical software such as R and G*Power.
Statistical Power Analysis and Errors
There are two types of errors; type 1 and type II errors. Type 1 error (false positive) is rejecting the null hypothesis when it is true, while type II error (false negative) is failing to reject the null hypothesis when it is false. The power of a test is randomly set at 80%, meaning if there is a difference, 20% is the probability of type II error occurring. Hence, the power of a test is the probability of successfully rejecting the null hypothesis when it is false (1-ᵦ). The value of the power of a test can be appropriate depending on the balance between the type 1 and type II error.
Factors Affecting Power Analysis
(a). Sample Size
Given a sample size, the statistical power increases as the size of the sample increases. A large sample size increases the statistical power thus reducing the chances of type II error occurring and detecting an effect of smaller size. A small sample size increases the individual variance and raises the type 1 error threshold.
(b). Effect Size
Effect size is the probability that a subject randomly selected from one group will have a higher score than a subject randomly selected from a different group. Effect size is conveyed as the standardized mean difference between two subjects. The null hypothesis and p-value provide evidence of effect size. Smaller effect sizes are not significant to the analysis since they are barely detected. In some instances, the small effect size can be detected if the study had a large sample size. A large effect size provides a strong statistical power (Breur, 2016). The variables chosen in a study have a direct effect on the output as they can be used to design and estimate the sample size for a related study in the future.
(c). Decision Criteria(α)
The decision about the standard of rejecting the null hypothesis must be made to define the threshold under which the study lies. It’s easier to reject the null hypothesis with a 0.05 significant level (α) rather than 0.01 or 0.1. A significant level of 0.05 has a 5% chance of type 1 error, hence the power of the test will be given by (1- α), the specificity of the test.
Summary
Researchers put more emphasis on the significance of the research and little on the power analysis. Power analysis is the probability of type II error occurring within a 5% threshold. Power analysis shows the effect of interest, its magnitude, and how it will affect the output of the research.
Factors such as sample size, effect size, and decision criterion affect the power analysis. Sample size and effect size are directly proportional to statistical power analysis. As sample size and effect size increase, the power analysis increases. Large sample size and effect size increases the probability of identifying the effect and its magnitude on the topic of research.
The standard significant level that can yield conclusive power analysis is 0.05. Power analysis should be incorporated more in future research so as to explain the effect and its magnitude. Power analysis will help design, plan future studies, and estimate the required sample sizes for similar studies in the future.